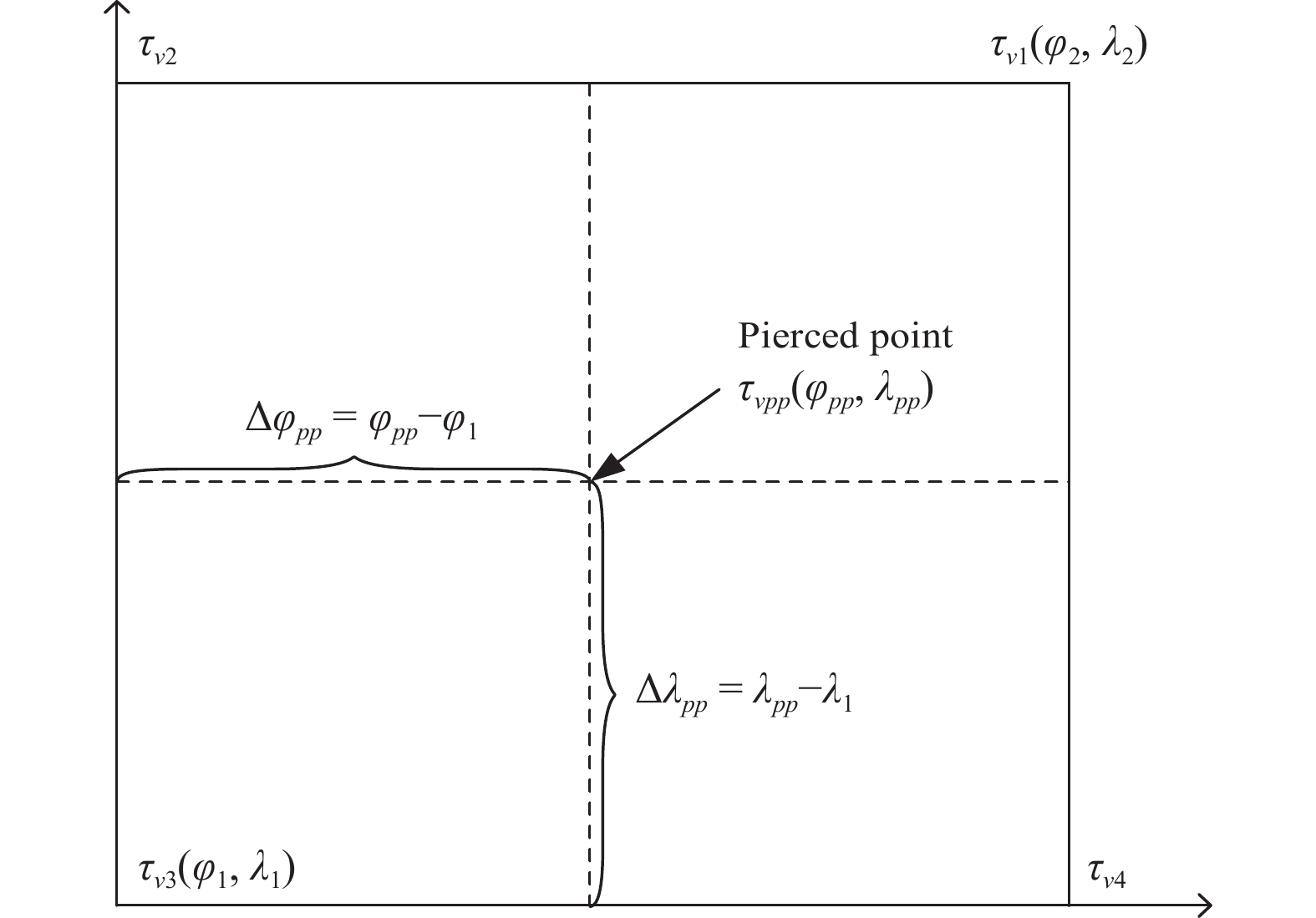
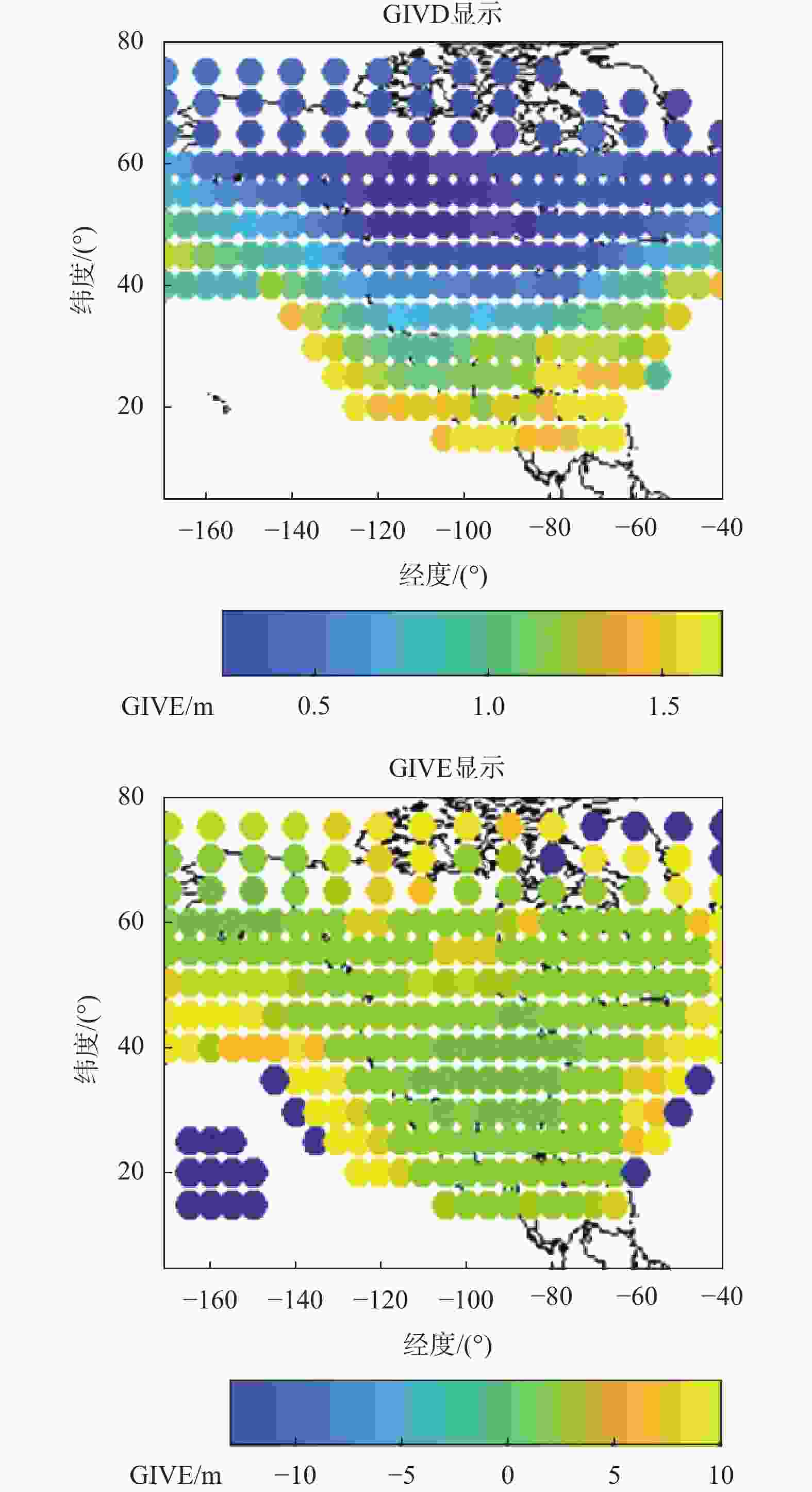
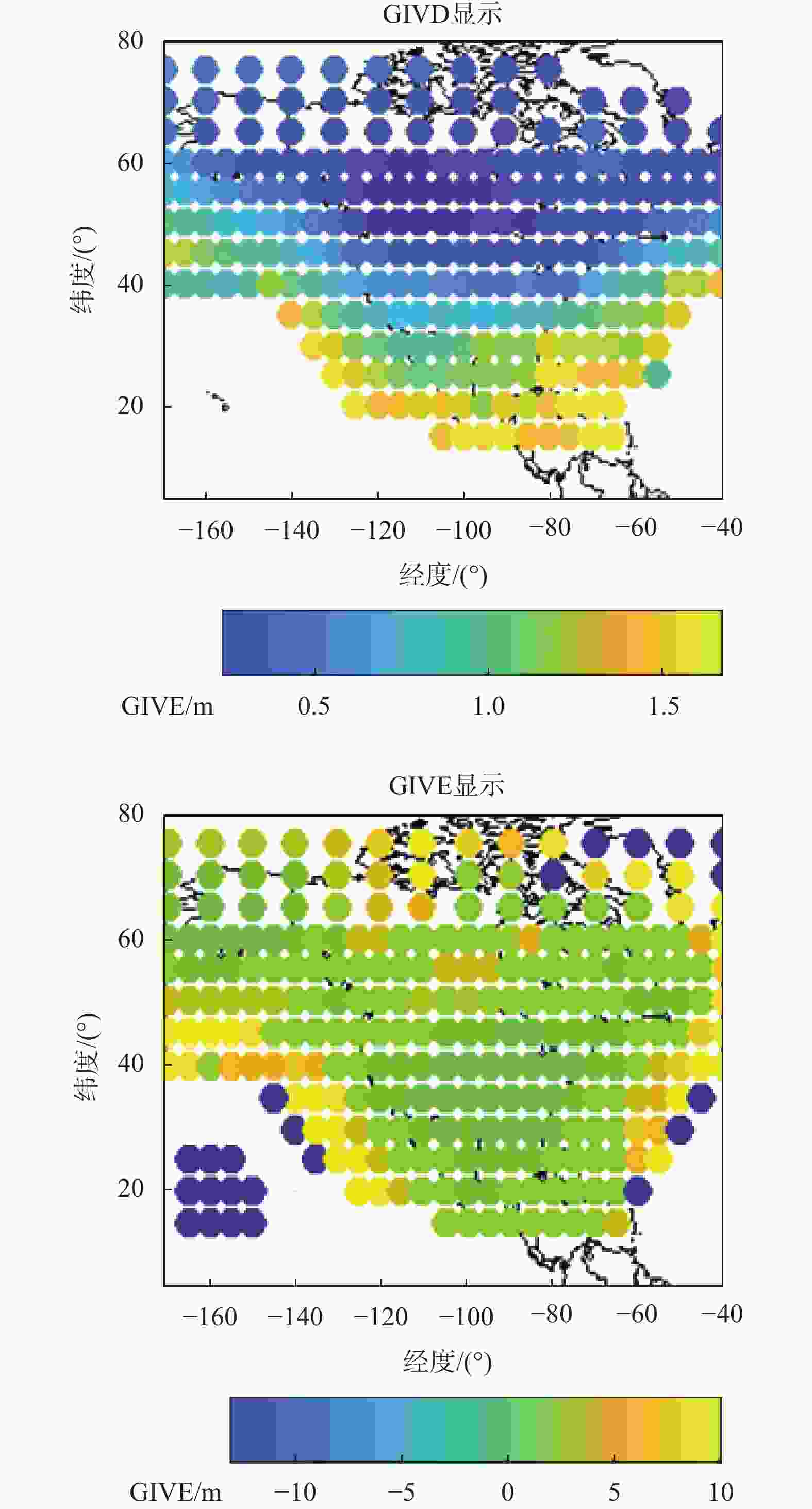
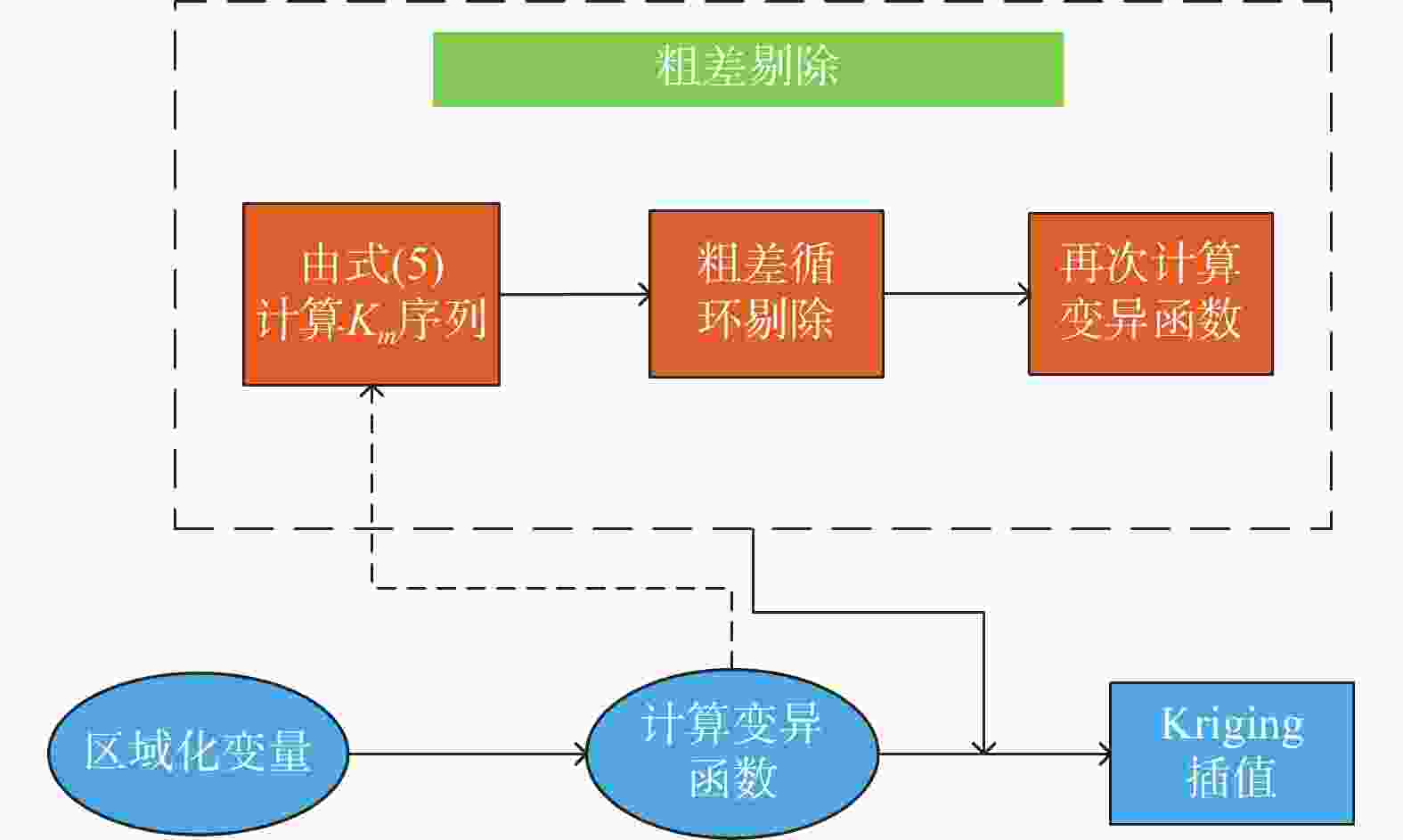
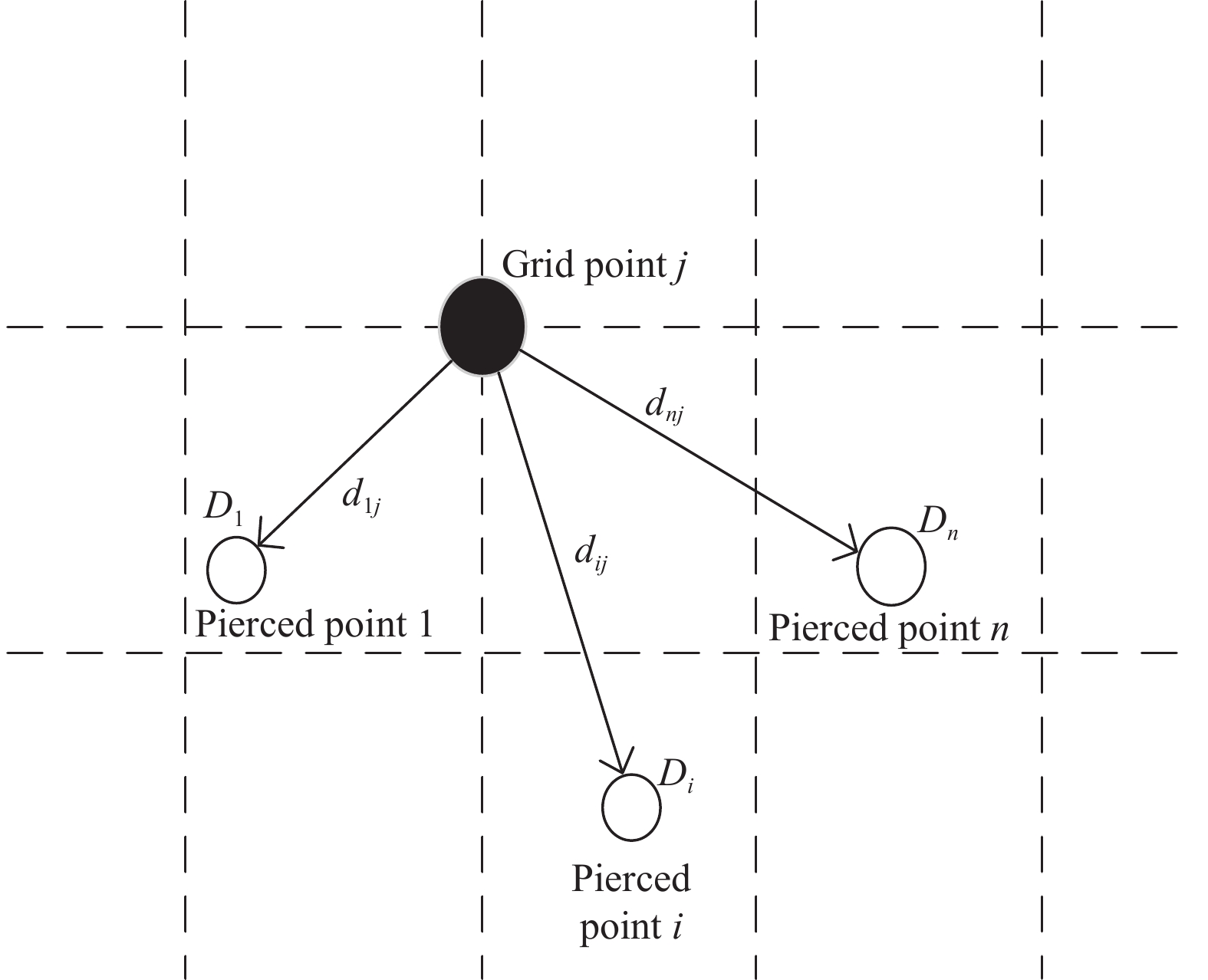
| [1] |
SAĞIR S, ATICI R, AKALIN A, et al. The assessment in terms of QBO of NeQuick 2 model[J]. Egyptian journal of remote sensing and space sciences, 2019, 22(1): 67-72. DOI: 10.1016/J.EJRS.2018.07.004
|
| [2] |
王宁波, 袁运斌, 李子申, 等. 不同NeQuick电离层模型参数的应用精度分析[J]. 测绘学报, 2017, 46(4): 421-429.
|
| [3] |
谢钢. GPS原理与接收机设计[M]. 北京: 电子工业出版社, 2009: 80-85.
|
| [4] |
SUN M F, LIU L, YAN W, et al. Performance analysis of BDS B1C/B2a PPP using different models and MGEX products[J]. Survey review, 2022, 55(89): 192-203. DOI: 10.1080/00396265.2022.2081013
|
| [5] |
袁运斌, 李敏, 霍星亮, 等. 北斗三号全球卫星导航系统全球广播电离层延迟修正模型(BDGIM)应用性能评估[J]. 测绘学报, 2021, 50(4): 436-447.
|
| [6] |
CHEN L, LI M, HU Z G, et al. Method for real-time self-calibrating GLONASS code inter-frequency bias and improvements on single point positioning[J]. GPS solutions, 2018, 22(4): 1-12. DOI: 10.1007/s10291-018-0774-2
|
| [7] |
PAN L, ZHANG X H, GUO F. Characterizing inter-frequency bias and signal quality for GLONASS satellites with triple-frequency transmissions[J]. Advances in space research, 2019, 64(7): 1398-1414. DOI: 10.1016/j.asr.2019.06.038
|
| [8] |
CAO X Y, LI J C, ZHANG S J, et al. Uncombined precise point positioning with triple-frequency GNSS singles[J]. Advance in space research, 2019, 63(9): 2745-2756. DOI: 10.1016/J.ASR.2018.03.030
|
| [9] |
陈少鑫. GPS三频电离层误差改正算法研究[D]. 淮南: 安徽理工大学, 2019.
|
| [10] |
金蕾, 匡翠林. 基于地磁场建模的电离层误差二阶项改正方法[J]. 大地测量与地球动力学, 2012, 32(6): 119-122.
|
| [11] |
张鹏. 全球格网电离层模型在单频精密单点定位中的应用研究[D]. 长春: 吉林大学, 2021.
|
| [12] |
张卓轩. 复杂电离层环境下的GLS机载接收机性能评估技术研究[D]. 天津: 中国民航大学, 2022.
|
| [13] |
于耕, 曲歌. 北斗格网电离层模型格网点计算方法研究[J]. 电子技术应用, 2017, 43(6): 15-18.
|
| [14] |
ROVIR-GARCIA A, JUAN J M, SANZ J, et al. Accuracy of ionospheric models used in GNSS and SBAS: methodology and analysis[J]. Journal of geodesy, 2016, 90(3): 229-240. DOI: 10.1007/s00190-015-0868-3
|
| [15] |
LOPEZ-MARTINEZ M, ALVAREZ J M, LORENZO J M, et al. SBAS/EGNOS for maritime[J]. Journal of marine science and engineering. 2020, 8(10): 764. DOI: 10.3390/JMSE8100764
|
| [16] |
刘钝, 李锐. 卫星导航增强中的电离层扰动影响研究——基于系统可靠性工程的视角[J]. 全球定位系统, 2023, 48(1): 3-13.
|
| [17] |
BANVILLE S, HASSEN E, WALKER M, et al. Wide-area grid-based slant ionospheric delay corrections for precise point positioning[J]. Remote sensing, 2022(14): 1073. DOI: 10.3390/rs14051073
|
| [18] |
朱永兴, 谭述森, 杜兰, 等. 顾及粗差影响的全球电离层克里金插值及精度分析[J]. 测绘学报, 2019, 48(7): 840-848.
|
| [19] |
朱永兴, 谭述森, 任夏, 等. GNSS全球广播电离层模型精度分析[J]. 武汉大学学报(信息科学版), 2020, 45(5): 768-775.
|
| [20] |
黄玲, 章红平, 徐培亮, 等. 中国区域VTEC模型Kriging算法研究[J]. 武汉大学学报(信息科学版), 2016, 41(6): 729-737.
|
| [21] |
汤俊, 高鑫, 李垠健, 等. 2018年8月磁暴期间北斗GEO卫星电离层TEC时空变化分析[J]. 测绘学报, 2022, 51(3): 317-326.
|
| [22] |
田睿, 董绪荣. 小波分解与Prophet框架融合的电离层VTEC预报模型[J]. 系统工程与电子技术, 2021, 43(3): 610-622.
|
| [23] |
WANG S, WANG D, SUN J R. Artificial neural network-based ionospheric delay correction method for satellite-based augmentation systems[J]. Remote sensing, 2022(14): 676. DOI: 10.3390/rs14030676
|
| [24] |
YASYUKEVICH Y V, ZATOLOKIN D, PADOKHIN A, et al. Klobuchar, NeQuick G, BDGIM, GLONASS, IRI-2016, IRI-2012, IRI-Plas, NeQuick2, and GEMTEC ionospheric models: a comparison in total electron content and positioning domains[J]. Sensors, 2023, 23(10): 1424-8220. DOI: 10.3390/s23104773
|
| [25] |
CHEN J P, HU X G, TANG C P, et al. SIS accuracy and service performance of the BDS-3 basic system[J]. Science China physics, mechanics and astronomy, 2020, 63(6): 269511. DOI: 10.1007/s11433-019-1468-9
|
| [26] |
郭睿, 黄张裕, 孙瑞, 等. 北斗三号BDGIM模型的适用性分析[J]. 海洋测绘, 2021, 41(4): 61-63.
|
| [27] |
YUAN Y B, WANG N B, LI Z S, et al. The BeiDou global broadcast ionospheric delay correction model (BDGIM) and its preliminary performance evaluation results[J]. Navigation, 2019, 66(1): 1-15. DOI: 10.1002/NAVI.292
|
| [28] |
CHENG L, GAO W G, BO S, et al. Development of BeiDou satellite-based augmentation system[J]. Navigation-journal of the institute of navigation, 2021, 68(2): 405-417. DOI: 10.1002/NAVI.422
|
| [29] |
WANG N B, LI Z S, YUAN Y B, et al. BeiDou Global Ionospheric delay correction model(BDGIM): performance analysis during different levels of solar conditions[J]. GPS solutions, 2021, 25(3): 1-13. DOI: 10.1007/s10291-021-01125-y
|
| [30] |
XI K W, WANG X Y. Higher order ionospheric error correction in BDS precise orbit determination[J]. Advances in space research, 2021, 67(12): 4054-4065. DOI: 10.1016/J.ASR.2021.02.002
|
| [31] |
朱永兴. 北斗系统全球电离层建模理论与方法研究[J]. 测绘学报, 2021, 50(5): 710.
|
| [32] |
SU K, JIN S G, JIANG J, et al. Ionospheric VTEC and satellite DCB estimated from single-frequency BDS observations with multi-layer mapping function[J]. GPS solutions, 2021, 25(2): 1-17. DOI: 10.1007/s10291-021-01102-5
|
| [33] |
ZHANG R, SONG W W, YAO Y B, et al. Modeling regional ionospheric delay with ground-based BeiDou and GPS observations in China[J]. GPS solutions, 2015, 19(4): 649-658. DOI: 10.1007/s10291-014-0419-z
|
| [34] |
YANG C, GUO J, GENG T, et al. Assessment and comparison of broadcast ionospheric models: NTCM-BC, BDGIM, and Klobuchar[J]. Remote sensing, 2020, 12(7): 1215. DOI: 10.3390/rs12071215
|
| [35] |
WU X L, HU X G, WANG G, et al. Evaluation of COMPASS ionospheric model in GNSS positioning[J]. Advances in space research, 2013(51): 959-968. DOI: 10.1016/J.ASR.2012.09.039
|
| [36] |
谢杰, 姚志成, 刘鑫昌, 等. 双频GPS信号仿真的电离层误差补偿模型研究[J]. 微计算机信息, 2012, 28(5): 133-135.
|
| [37] |
WANG X L, LI Y F. Study on adaptability of GPS ionospheric error correction models[J]. Aircraft engineering and aerospace technology:an international journal, 2009, 81(4): 316-322. DOI: 10.1108/00022660910967309
|
| [38] |
刘宸, 刘长建, 冯绪, 等. 适用于不同尺度区域的Klobuchar-like电离层模型[J]. 测绘学报, 2016, 45(S2): 54-63.
|
| [39] |
ZHANG Q, LIU Z Y, HU Z G, et al. A modified BDS Klobuchar model considering hourly estimated night-time delays[J]. GPS solutions, 2022, 26(2): 1-13. DOI: 10.1007/s10291-022-01236-0
|
| [40] |
WANG N B, LI Z S, YUAN Y B, et al. Ionospheric correction using GPS Klobuchar coefficients with an empirical night-time delay model[J]. Advance in space research, 2019, 63(2): 886-896. DOI: 10.1016/J.ASR.2018.10.006
|
| [41] |
杨玲, 周春元, 苏小宁, 等. 附加IRI模型约束的全球电离层建模及定位精度分析[J]. 同济大学学报(自然科学版), 2021, 49(11): 1606-1613.
|
| [42] |
ZHU W, CHEN J Y, ZHANG Q, et al. Mapping of high-spatial-resolution three-dimensional electron density by combing of full-polarimetric SAR and IRI model[J]. Frontiers in earth science, 2020(8): 181. DOI: 10.3389/feart.2020.00181
|
| [43] |
MONTENBRUCK O, RODRIGUEZ B G. NeQuick-G performance assessment for space applications[J]. GPS solutions, 2019, 24(1): 1-12. DOI: 10.1007/s10291-019-0931-2
|
| [44] |
KIM J, KIM M. NeQuick G model based scale factor determination for using SBAS ionosphere corrections at low earth orbit[J]. Advanced in space research, 2020, 65(5): 1414-1423. DOI: 10.1016/j.asr.2019.11.038
|
| [45] |
ARAGON A, ZURN M, ROVIRA-GARCIA A. Galileo ionospheric correction algorithm: an optimization study of NeQuick-G[J]. Radio science, 2020, 54(11): 1156-1169. DOI: 10.1029/2019RS006875
|
| [46] |
TIAN Y, LI S H, SHEN H, et al. Comparative analysis of BDGIM, NeQuick-G, and Klobuchar ionospheric broadcast models[J]. Astrophysics and space science, 2022, 367(8): 78. DOI: 10.1007/s10509-022-04109-7
|
| [47] |
CIEĆKO A, GRUNWALD G. Klobuchar, NeQuick G, and EGNOS ionospheric models for GPS/EGNOS single-frequency positioning under 6-12 september 2017 space weather events[J]. Applied sciences-basel, 2020, 10(5): 78. DOI: 10.3390/app10051553
|
| [48] |
吴显兵, 阮仁桂. 伽利略电离层改正模型的精度对比分析[J]. 测绘科学, 2015, 40(5): 17-20.
|
| [49] |
韩玲, 王解先, 柳景斌. NeQuick模型算法研究及性能比较[J]. 武汉大学学报(信息科学版), 2018, 43(3): 464-470.
|
| [50] |
WANG C, ZHANG T, FAN L, et al. A simplified worldwide ionospheric model for satellite navigation[J]. IEEE transactions on aerospace and electronic systems, 2022, 58(1): 391-405. DOI: 10.1109/taes.2021.3103259
|
| [51] |
韩玲, 王解先, 陈艳玲, 等. 利用GNSS数据结合NeQuick模型优化磁暴期F2层临界频率参数估计[J]. 测绘学报, 2020, 49(1): 14-23.
|
| [52] |
CIRO G, ANTONIO A, SALVATORE G. Neustrelitz total electronic content model for galileo performance: a position domain analysis[J]. Sensors, 2022, 26(3): 3766. DOI: 10.3390/s23073766
|
| [53] |
肖勇. 高纬度区域GNSS多系统电离层建模及其精度评估[J]. 全球定位系统, 2023, 48(3): 33-38.
|
| [54] |
KIM B C, TININ M V. Potentialities of multi-frequency ionospheric correction in Global Navigation Satellite Systems[J]. Journal of geodesy, 2011, 85(3): 159-169. DOI: 10.1007/S00190-010-0425-Z
|
| [55] |
陈正生, 张清华, 李林阳, 等. 电离层延迟变化自模型化的载波相位平滑伪距算法[J]. 测绘学报, 2019, 48(9): 1107-1118.
|
| [56] |
BOLLA P, WON J H. Performance analysis of geometry-free and ionosphere-free code-carrier phase observation models in integer ambiguity resolution[J]. IET radar and navigation, 2018, 12(11): 1313-1319. DOI: 10.1049/IET-RSN.2018.5036
|
| [57] |
李宏宇. 多模多频非差非组合精密单点定位方法研究[D]. 哈尔滨: 哈尔滨理工大学, 2021.
|
| [58] |
高爽. BDS/GNSS多参考站多模多频高精度定位技术研究[D]. 哈尔滨: 哈尔滨工程大学, 2020.
|
| [59] |
GAO W, GAO C, PAN S. A method of GPS/BDS/GLONASS combined RTK positioning for middle-long baseline with partial ambiguity resolution[J]. Empire survey review, 2015, 49(354): 212-220. DOI: 10.1179/1752270615Y.0000000047
|
| [60] |
李磊, 徐爱功, 祝会忠, 等. 长距离网络RTK基站间整周模糊度的快速解算[J]. 测绘科学, 2014, 39(10): 22-25.
|
| [61] |
王生朝. 北斗三频模糊度解算方法研究[D]. 徐州: 中国矿业大学, 2015.
|
| [62] |
GE Y L, DING S, QIN W J, et al. Performance of ionospheric-free PPP time transfer models with BDS-3 quad-frequency observations[J]. Measurement, 2020, 160: 107836. DOI: 10.1016/j.measurement.2020.107836
|
| [63] |
李博峰, 葛海波, 沈云中. 无电离层组合、Uofc和非组合精密单点定位观测模型比较[J]. 测绘学报, 2015, 44(7): 734-740.
|
| [64] |
YAN Z B, ZHANG X H. Assessment of the performance of GPS/Galileo PPP-RTK convergence using ionospheric corrections from networks with different scales[J]. Earth, planets and space, 2022, 74(1): 1-19. DOI: 10.1186/s40623-022-01602-9
|
| [65] |
YIN X, CHAI H Z, XU W B, et al. Realization and evaluation of real-time uncombined GPS/Galileo/BDS PPP-RTK in the offshore area of China’s Bohai Sea[J]. Marine geodesy, 2022, 45(6): 577-594. DOI: 10.1080/01490419.2022.2057628
|
| [66] |
LI P, CUI B B, HU J H, et al. PPP-RTK considering the ionosphere uncertainty with cross-validation[J]. Satellite navigation, 2022, 3(1): 1-13. DOI: 10.1186/s43020-022-00063-5
|
| [67] |
ZHANG X H, REN X D, CHEN J, et al. Investigating GNSS PPP-RTK with external ionospheric constraints[J]. Satellite navigation, 2022, 3(6): 1-13. DOI: 10.1186/s43020-022-00071-5
|
| [68] |
宋伟伟, 何成鹏, 辜声峰. 不同纬度区域电离层增强PPP-RTK性能分析[J]. 武汉大学学报(信息科学版), 2021, 46(2): 1832-1842.
|
| [69] |
CAI C S, LIU G, YI Z H, et al. Effect analysis of high-order ionospheric corrections on quad-constellation PPP[J]. Measurement science and technology, 2019, 30(2): 1-16. DOI: 10.1088/1361-6501/aaf555
|
| [70] |
黄令勇, 吕志平, 刘毅锟, 等. 三频BDS电离层延迟改正分析[J]. 测绘科学, 2015, 40(3): 12-15.
|
| [71] |
LI J L, YANG Y X, HE H B, et al. Benefits of BDS-3 B1C/B1I/B2a triple-frequency signals on precise positioning and ambiguity resolution[J]. GPS solutions, 2020, 24(4): 1-10. DOI: 10.1007/s10291-020-01016-8
|
| [72] |
YAN Z B, ZHANG X H. The performance of three-frequency GPS PPP-RTK with partial ambiguity resolution[J]. Atmosphere, 2022, 13(7): 1014. DOI: 10.3390/atmos13071014
|
| [73] |
陈少鑫, 徐良骥. GPS 电离层折射误差的三阶三频改正模型及精度分析[J]. 测绘通报, 2018(12): 10-14.
|
| [74] |
MARQUES H, MONICO G, AQUINO M. RINEX_HO: second-and third-order ionospheric corrections for RINEX observation files[J]. GPS solutions, 2011, 15(3): 305-314. DOI: 10.1007/s10291-011-0220-1
|
| [75] |
HAMMER M D, COX G A, BROWN W J. Geomagnetic virtual observatories: monitoring geomagnetic secular variation with the swarm satellites[J]. Earth plants and space, 2021, 73: 1-22. DOI: 10.1186/s40623-021-01357-9
|
| [76] |
TU R, ZHANG P F, ZHANG R, et al. Modeling and performance analysis of precise time transfer based on BDS triple-frequency un-combined observations[J]. Journal of geodesy, 2019, 93(6): 837-847. DOI: 10.1007/s00190-018-1206-3
|
| [77] |
LI D H, MI J Z, CHENG P F, et al. A cycle slip repair method against ionospheric effects and observations noises for BDS triple-frequency undifferenced phases[J]. Sensors, 2020, 20(10): 1-21. DOI: 10.3390/s20102819
|
| [78] |
ZHANG R C, GAO C F, WANG Z B, et al. Ambiguity resolution for long baseline in a network with BDS-3 quad-frequency ionosphere-weighted model[J]. Remote sensing, 2022, 14(7): 1-18. DOI: 10.3390/rs14071654
|
| [79] |
AN X D, MENG X L, CHEN H, et al. Modelling global ionosphere based on multi-constellation GNSS observations and IRI model[J]. Remote sensing, 2020, 12(3): 1-19. DOI: 10.3390/rs12030439
|



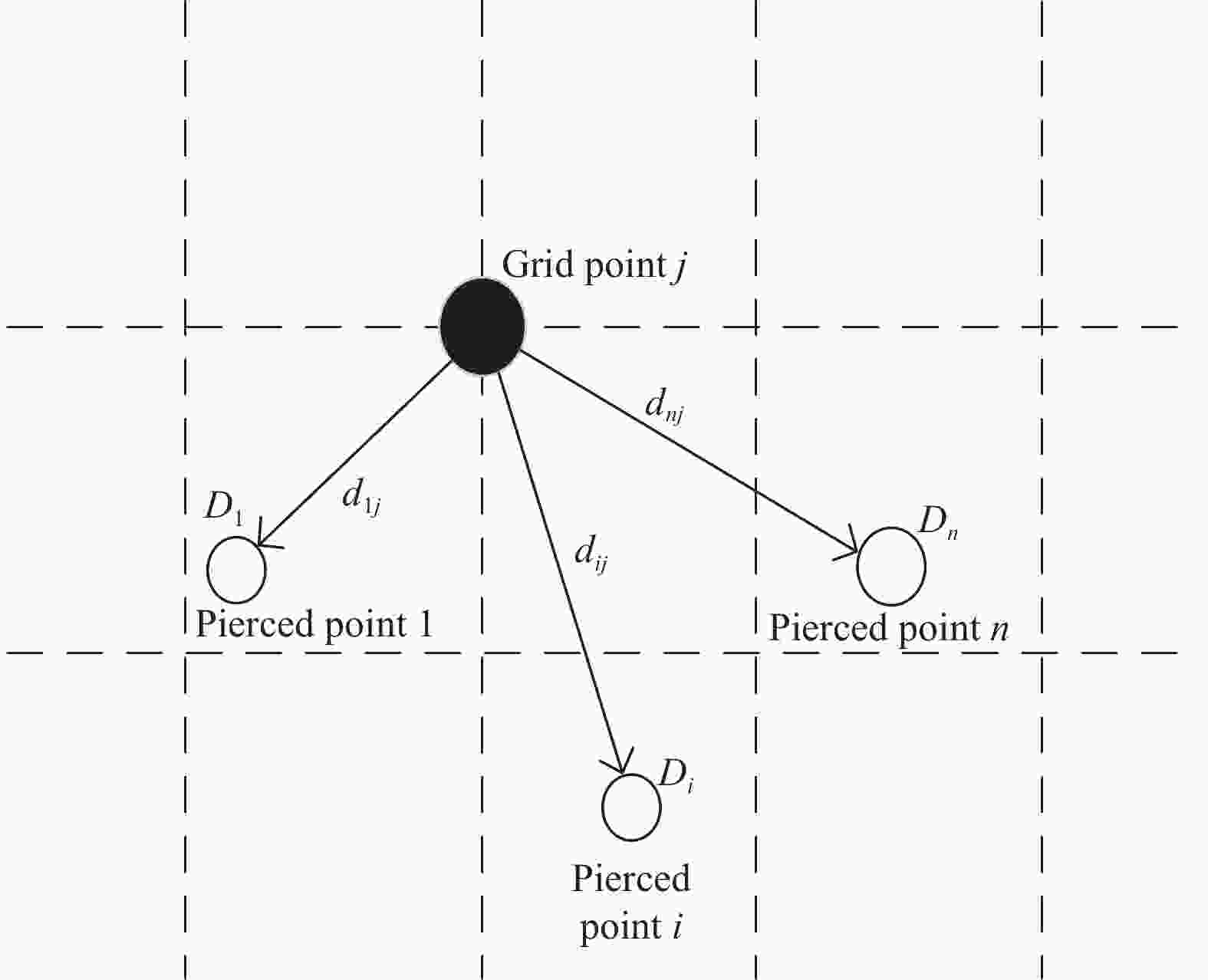
 下载:
下载: